In what follows, 'logic' means "a thing is not what it is not." Imagine if logic did not exist. That fact would be an example of logic. So, logic exists.
Imagine if nothing at all existed. Actually, that's not possible. When we say "nothing" we always mean "not a particular thing". For example, "nothing in my hand" means "not the kind of thing you might be interested in" since my hand does in fact contain air.
Even the vacuum of empty space contains quarks popping in and out of existence. Even a mathematical empty set contains something: a logical concept of empty. If it did not then the computer would crash every time it met an empty set: but this "nothing" has something: the implication of its surrounding structure.
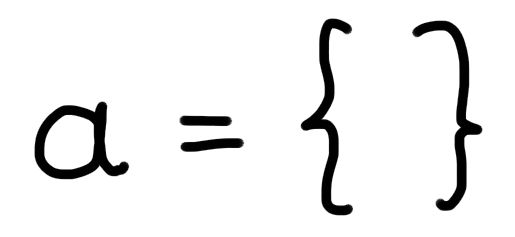
When we imagine absolute nothing - when we try to empty our minds - we are really imagining ignorance.

Another way to think of nothing is to imagine a world of zero size with zero content. Such a world does in fact exist: an infinite number of them exist in the palm of my hand. And the world goes on around them as usual.
But however we imagine nothing, it is a concept: "not something". So, concepts exist, whatever they are. But "not something" implies two concepts: "something" and "not". So, two concepts exist.

"Two" is another concept: so we have three concepts.

Continue this reasoning and we have "four", "five", etc. So, numbers exist.

Apply that same reasoning to numbers as a group, and we have a concept "some number". We can then do the same with them, having "one number", "two of that number" and so on.
Pretty soon we find ourselves with dimensions (numbers made of numbers), variables, the concept of multiplication (two of something is the same concept no matter what that something is), and so on. In short, the whole of mathematics appears before your eyes.
Theoretical physicists merely give names to dimensions (let's call this one "space" and this one "time") and work out the equations that fit the universe that we observe. Other universes would have different equations.

Every point in dimensional space (e.g. "x=3, y=4") is connected to every other point by different equations: e.g. (3,4) + (1,1) = (4,5). Some equations require more steps than others: they are more difficult. We could call the dimensions "space-time" and the total number of steps in an equation could be called "difficulty" or "energy".

To move between adjacent points requires little energy, unless we take a very indirect route. We could even calculate the steps back to the zero point ("Big Bang" or before). We can then find then the energy needed to create a very different universe. So every universe is connected, all part of the same number lines.
"But wait", you say, "how can numbers make a physical universe?" Physical just means force is involved. E.g. move your hand to a wall, your hand is stopped by the wall, you feel a force.
Force just means a mass changes speed. (Force equals mass times acceleration, F = m a). Mass is just a number: it only has meaning because it changes speed when other masses are near. You experience speed changing simply because you (the neurons in your brain) are part of the mathematical universe. So force is just time and space, just numbers.

After making the page about why things exist, I was delighted to learn that the ancient philosphers came to similar conclusions:
"That the monad [the indivisible origin: what I call "something"] was the beginning of everything. From the monad proceeds an indefinite duad [what I call "not something"], which is subordinate to the monad as to its cause. That from the monad and the indefinite duad proceed numbers. And from numbers signs. And from these last, lines of which plane figures consist. And from plane figures are derived solid bodies. And from solid bodies sensible bodies, of which last there are four elements; fire, water, earth, and air." (From the 3rd century text "Lives and Opinions of Eminent Philosophers")
Regarding fire, water, earth and air" as fundamental states of all matter, we now call the fundamental states "plasma, liquid, solid and gas". Early chemists categorised all plasma under the heading "fire", all liquids under the heading "water", all solids under the heading "earth", and all gases under the heading "air".
In conclusion, the Pythagoreans were right. They taught that "The principles of mathematics are the principles of all things" (See Aristotle, Metaphysics 1:5).